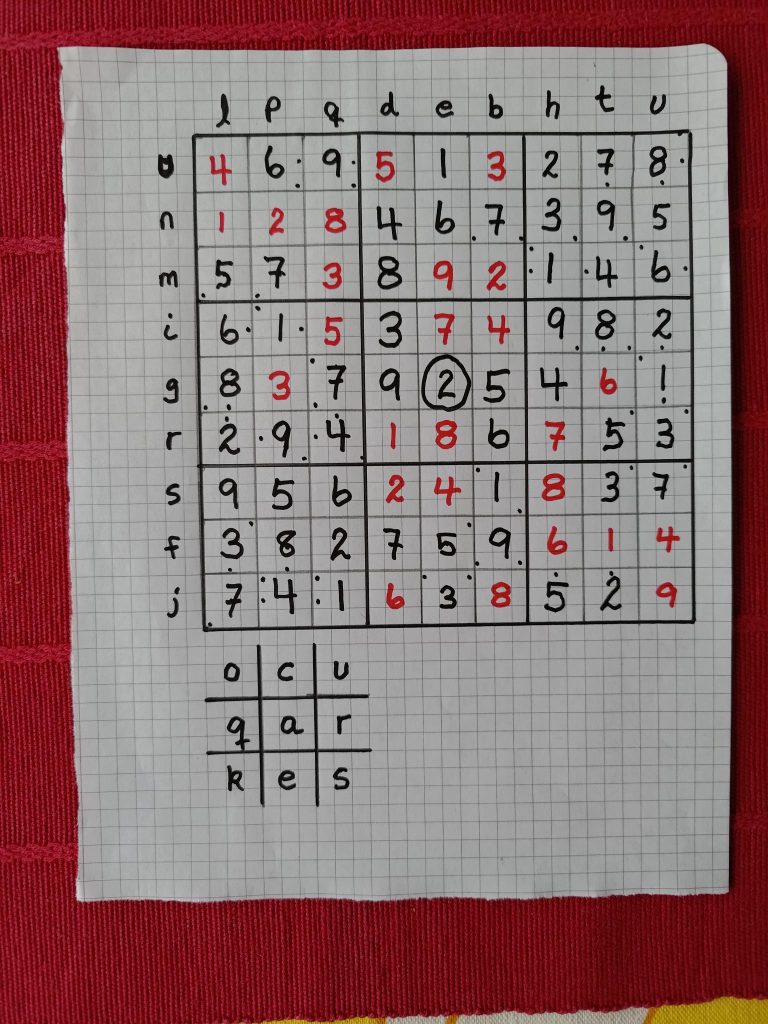
Typically, I begin a weblog post by writing its title. Nobody has ever accused me of being a good speller. I am better now than I was in elementary school, and increasingly better because the text processing programs I use habitually mark misspelled words by underlining them in red. The good news is that I know the consonants making up the title word here, and their order: s – d – k. In addition, I know, that this title only had a combination of o’s and u’s as vowels, but I can not remember how many of each, or their placement. After all, it was several minutes since I had closed a book with 500 such puzzles, to recreate a puzzle. Thus I began with a known incorrect spelling Sodoko then replaced the o’s with u’s until the red lines disappeared.
Compared to spelling, Sudoku is logical. There is a single objective, and many aids to assist the person achieving the game’s goal. Wikipedia tells us that in classic Sudoku, the objective is to fill a 9 × 9 grid with digits so that each column, each row, and each of the nine 3 × 3 subgrids (referred to as felt = field, in some languages) that compose the grid contains all of the digits from 1 to 9.
In puzzle books and apps, some numbers are printed. In the example above, I have written these in red, so that the task is to find the missing numbers, which are printed in black. In this example, there are 26 numbers printed in red, so my task is to find the remaining 81 – 26 = 55 numbers.
My game involves four deviant actions using non-conventional markings. The first is dot notation. I allow myself to mark up to two potentially correct answers in any square. If one of those numbers is invalidated somewhere, I can add yet another potential answer. I divide each square into a grid of nine dots. The top three represents numbers: 1 – 2 – 3, the middle: 4 – 5 – 6, and the bottom 7 – 8 – 9. Eight of the nine dots are along the margins, only 5 is in the middle of the square. When I know with certainty the correct answer, I write it in the square.
Second, I draw a grid of 2 vertical and 2 horizontal lines representing the Sudoku subgrid layout. This is because, I note the order in which I complete each horizontal or vertical line, as well as the subgrids. I order these by writing a lower-case letters along the top, left side and inside the subgrid, starting with a.
Third, the very first time in each game that I put an answer in a square, I ring the answer, so that I can see where I started.
Fourth, sometimes I am left with a situation where I have no clear answer to write, but two potential answers in one square. I then change one of the potential answer dots in that square to a small asterisk (which I have just learned is not spelled asterix), then play through marking the results in other squares with additional x’s, as required. At some point the sequence of answers will either show itself to be correct or incorrect. If it is correct then I mark the asterisk square, and all of the x squares, with the correct numerical values. If it is incorrect, then I go back and try with the remaining number, which should be correct. The reason for using an asterisk, is that it helps me to remember the square where I started!
One would think that potentially there would be a need for 9 + 9 + 9 = 27 lower-case letters to mark complete entries in rows, columns and sub-grids. The first unit completed is marked with a, followed by b and onwards through the alphabet. Often, one completes two or more of the units simultaneously. In such a situation, the same letter can be used to mark up to three units: a vertical line, a horizontal line and a subgrid. The last entry, in particular, does this. Thus, the last letter I have used is no later in the alphabet than y, but it is often as low as a q. In the example shown, the last letter used was “u”. The row and column marked with u, indicate that the last number put into the grid was 8, at the top right of the grid.
Undoubtedly, many readers are wondering what I do with all of this extra information? The honest answer is, nothing. It is just my approach to playing the game of Sudoku. I play it on a daily basis, so that my brain does not degenerate completely. I also work with Duolingo on a daily basis for the same reason.
This particular puzzle was #447, randomly taken from the book Mega Sudoku (2023), published by Zebra AS. It contains 500 puzzles that are assigned a level: * = beginner (1-40); ** = easy (41 – 190); *** = captivating (191 – 340); **** = challenging (341 – 476); ***** = expert (477 – 500). I began working on this book 2023-07-20, and had completed all the 3 and 4 star puzzles about a year later, in addition to a few 2 and 5 star puzzles. Some day, this book will be recycled to someone/ anyone wanting to start playing Sudoku.
This was my last book of Sudoku puzzles, and my last opportunity to use the methods described here. I have now gone over to using an app on my hand-held device. The main reason has to do with greed. My previous Mega Sudoku books provided 1 000 puzzles, for approximately the same price that 500 puzzles cost in 2023. The app was acquired without any expense. It provides many of the same benefits I appreciate on the paper edition, such as levels of play. It also provides a very large, but not unlimited, number of games. For insights into the mathematics involved, Wikipedia has provided an article about the mathematics of Sudoku.
Origins
Sudoku’s origins can be traced back in time to Chinese culture and the Magic Square puzzle. This involves arranging numbers in a square grid such that each row, column, and diagonal adds up to the same sum. The earliest known Magic Squares is from the period of Emperor Yu (ca. 2200 BCE) who allegedly created a 3×3 Magic Square called the Lo Shu Square. The Lo Shu Square is said to have been discovered on the back of a turtle emerging from the Yellow River. This 3×3 grid contains the numbers 1 to 9, arranged in a way that the sum of each row, column, and diagonal equals 15, as shown below.

There are other magic squares. They all arranges numbers in a grid without repetition and having certain sums. With larger grids came more complex rules.
Modern Sudoku is not based on ancient Chinese number placement puzzles. It emerged in the 18th century and gained popularity in Switzerland and Japan. In 18th century Switzerland, Leonhard Euler (1707–1783) studied combinatorics. A Latin square is an arrangement of numbers or symbols in a grid, such that each element appears exactly once in each row and each column. Developments here provided a foundation for the logical reasoning and patterns involved in solving this type of puzzle.
In the late 1970s, Howard Garns (1905 – 1989) developed the modern version of Sudoku, Number Place, with a 9×9 grid with empty spots to fill using a set of simple rules.
Maki Kaji ( 1951 – 2021) was president of Nikoli, a Japanese puzzle manufacturer. He introduced the game to a broader audience when a Number Place puzzle first appeared in Monthly Nikolist in 1984-04 as Sūji wa dokushin ni kagiru (数字は独身に限る) = the digits must be single, with the Japanese dokushin = unmarried person. The name was later abbreviated to Sudoku (数独), which is a registered trademark in Japan.
The global spread of Sudoku can be attributed to Wayne Gould (1945 – ). In 1997 he found a Sudoku book in a bookstore in Tokyo. He then spent 6 years developing a computer program, known as Pappocom Sudoku, that could mass-produce puzzles for the global market. He convinced The Times to publish Sudoku as a regular feature, starting in 2004.
As Sudoku gained popularity puzzle creators introduced variations to add complexity and excitement to the game. Samurai Sudoku combines five overlapping grids, each with its own unique solution. Killer Sudoku introduced mathematical operations and sum constraints, challenging players to calculate and place numbers strategically. Interactive Sudoku apps create an endless variety of Sudoku puzzles, at different levels of difficulty. Some allow players worldwide to compete with each other. This has transformed Sudoku away from paper to internet based screens, complete with social media interaction.
Sudoku engages the brain in logic-based problem-solving. It is claimed that regular playing can improve memory, concentration and critical thinking skills. Problem solving involves structured thinking and the development of a more analytical mindset. It is also claimed that Sudoku can also be used in cognitive therapy. It is used to help patients with memory loss and other cognitive impairments.
For those wanting to compete with others, the World Puzzle Federation (founded in 1992) has hosted a World Sudoku Championship since 2006. Guinness World Records include: the fastest time to complete a Sudoku puzzle; the most puzzles solved in a certain time frame; and, the largest Sudoku grid ever created.

